Suppose that in winter the daytime temperature in a certain office building is maintained at 70°F. The heating
is shut off at 10 P.M. and turned on again at 6 A.M. On a certain day the temperature inside the building at 2 A.M.
was found to be 65°F. The outside temperature was 50°F at 10 P.M. and had dropped to 40°F by 6 A.M. What
was the temperature inside the building when the heat was turned on at 6 A.M.?
Physical information. Experiments show that the time rate of change of the temperature T of a body B (which
conducts heat well, for example, as a copper ball does) is proportional to the difference between T and the
temperature of the surrounding medium (Newton’s law of cooling).
加熱は午後10時に停止します。午前6時に再びオンになりましたある日、建物内の温度は午前2時です。
65°Fであることがわかった。外気温は午後10時で50°Fでした。午前6時までに華氏40度まで下がりました。午前6時に暖房がオンになったときの建物内の温度は何でしたか?
物理的な情報。実験では、体Bの温度Tの時間変化率(
例えば、銅ボールのように熱をよく伝導します)は、Tと
周囲の媒体の温度(ニュートンの冷却の法則)。
Solution. Step 1. Setting up a model. Let T(t)be the temperature inside the building and TA the outside
temperature (assumed to be constant in Newton’s law). Then by Newton’s law,
(6)dTdt=k(T–TA)
Such experimental laws are derived under idealized assumptions that rarely hold exactly. However, even if a
model seems to fit the reality only poorly (as in the present case), it may still give valuable qualitative information.
To see how good a model is, the engineer will collect experimental data and compare them with calculations
from the model.
温度(ニュートンの法則で一定と仮定)。次に、ニュートンの法則により、(6)dTdt=k(T–TA)
そのような実験法則は、正確に保持されることはめったにない理想的な仮定の下で導き出されます。ただし、たとえ
モデルは現実にあまり適合していないように思われ(現在の場合のように)、それでも価値ある定性的情報を提供する可能性があります。
モデルの良さを確認するために、エンジニアは実験データを収集し、計算と比較します
Step 2. General solution. We cannot solve (6) because we do not know TA, just that it varied between 50°F
and 40°F, so we follow the Golden Rule: If you cannot solve your problem, try to solve a simpler one. We
solve (6) with the unknown function TA replaced with the average of the two known values, or 45°F. For physical
reasons we may expect that this will give us a reasonable approximate value of T in the building at 6 A.M.
For constant (or any other constant value) the ODE (6) is separable. Separation, integration, and
taking exponents gives the general solution
dTT–45=kdt, ln|T−45|=kt+c∗,T(t)=45+cekt (c=ec∗)
そして華氏40度なので、ゴールデンルールに従います。問題を解決できない場合は、より単純な問題を解決してください。私達
(6)未知の関数TAを2つの既知の値の平均、または45°Fに置き換えて解きます。物理的に
これにより、午前6時の建物のTの適切な近似値が得られると予想される理由。
定数TA = 45(または他の定数値)の場合、ODE(6)は分離可能です。分離、統合、および
指数を取ると一般的な解が得られますdTT–45=kdt, ln|T−45|=kt+c∗,T(t)=45+cekt (c=ec∗)
Step 3. Particular solution. We choose 10 P.M. to be Then the given initial condition is and
yields a particular solution, call it TP. By substitution,
T(0)=45+ce0=70, c=70–45=25,TP(t)=45+25ekt
特定のソリューションが得られる場合、TPを呼び出します。置換により、
T(0)=45+ce0=70, c=70–45=25,TP(t)=45+25ekt
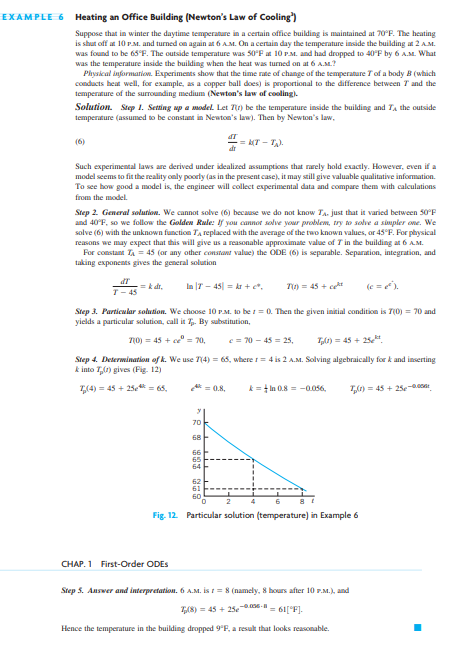
Step 4. Determination of k. We use T(4) = 65 , where t = 4 is 2 A.M. Solving algebraically for k and inserting
k into gives (Fig. 12)
TP(4)=45+25e4k=65, e4k=0.8,k=\frac{1}{4}ln0.8 = -0.056,T_{P}(t) = 45 + 25e^{-0.056t} $
k intoが与える(図12)TP(4)=45+25e4k=65, e4k=0.8,k=\frac{1}{4}ln0.8 = -0.056,T_{P}(t) = 45 + 25e^{-0.056t} $
Step 5. Answer and interpretation. 6 A.M. is t = 8(namely, 8 hours after 10 P.M.), and
TP(8)=45+25e−0.056・8=61[℉]
Hence the temperature in the building dropped 9°F, a result that looks reasonable.
数学を学ぶ意義:https://livemyself.com/archives/19038
[おすすめ図書]
上記リンクから購入した方は、内容の電子データを差し上げます。
ブログ管理人に気軽にメッセージしてください。