radioactive exponential decay

Given an amount of a radioactive substance, say, 0.5 g (gram), find the amount present at any later time.

PhysicalInformation.

Experiments show that at each instant a radioactive substance decomposes—and is thus decaying in time—proportional to the amount of substance present.

 

 

放射性物質の量、たとえば0.5 g（グラム）が与えられたら、後で存在する量を見つけます。

物理情報。

実験は、放射性物質が各瞬間に、存在する物質の量に比例して分解することを示しています。

 

 

Step 1. Setting up a mathematical model of the physical process. Denote by y(t) the amount of substance still present at any time t.
By the physical law , the time rate of change y'(t) = dy/dt is proportional to y (t) this gives the first-order ODE.

(6) dy/dt = -kt

where the constant k is positive , so that , because of the minus , we do get decay.
The value of k is known from experiments for various radioactive substances (e.g., k=1.4/10^-11sec^-1 approximately , for radium )

ステップ1.物理プロセスの数学モデルを設定します。いつでも存在する物質の量をy（t）で示します。 物理法則により、時間変化率y ‘（t）= dy / dtはy（t）に比例し、これにより1次ODEが得られます。 dy / dt = -kt ここで、定数kは正であるため、マイナスのため、減衰します。 kの値は、さまざまな放射性物質の実験から知られています（例：k = 1.4 / 10 ^ -11sec ^ -1、ラジウムの場合）

Now the given initial amount is 0.5 g, and we can call the corresponding instant Then we have the
initial condition This is the instant at which our observation of the process begins. It motivates
the term initial condition (which, however, is also used when the independent variable is not time or when
we choose a t other than ). Hence the mathematical model of the physical process is the initial value problem

与えられた初期量は0.5 gであり、対応するインスタントを呼び出すことができます
初期条件これは、プロセスの観察が始まる瞬間です。やる気を起こさせる
初期条件という用語（ただし、独立変数が時間でない場合や、
）以外のtを選択します。したがって、物理プロセスの数学的モデルは初期値です問題

(7) dy/dt = -kt , y(0) = 0.5.

Step 2. Mathematical solution. As in (B) of Example 3 we conclude that the ODE (6) models exponential decay
and has the general solution (with arbitrary constant c but definite given k)

ステップ2.数学的ソリューション。例3の（B）のように、ODE（6）は指数関数的減衰をモデル化すると結論付けます
そして、一般的な解を持っています（任意の定数cで、kが与えられた場合に限定されます）

(8) y(t) = ce^-kt

We now determine c by using the initial condition. Since y(0) = c from (8), this gives y(0) = c = 0.5. Hence
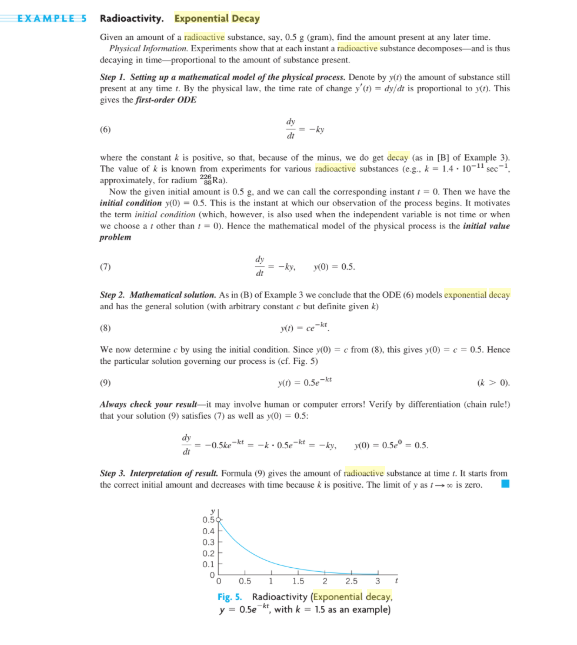
the particular solution governing our process is (cf. Fig. 5)

(9) y(t) = 0.5 e^-kt

ここで、初期条件を使用してcを決定します。 （8）からy（0）= cであるため、これによりy（0）= c = 0.5が得られます。したがって
プロセスを管理する特定のソリューションは次のとおりです（図5を参照）。

Always check your result—it may involve human or computer errors! Verify by differentiation (chain rule!)
that your solution (9) satisfies (7) as well as y(0) = 0.5:

dy/dt = -0.5ke^-kt = -k/0.5e^-kt = -ky , y(0) = 0.5e^0 = 0.5.

Step 3. Interpretation of result. Formula (9) gives the amount of radioactive substance at time t. It starts from
the correct initial amount and decreases with time because k is positive. The limit of y as t →　∞　 is zero.

ステップ3.結果の解釈。式（9）は、時刻tにおける放射性物質の量を示しています。から始まる
正しい初期量であり、kが正であるため時間とともに減少します。 t→∞としてのyの制限はゼロです。

 

 

 

放射性指数減衰 (https://ja.wikipedia.org/wiki/%E6%8C%87%E6%95%B0%E9%96%A2%E6%95%B0%E7%9A%84%E6%B8%9B%E8%A1%B0)

数学を学ぶ意味：https://livemyself.com/archives/19038

 

[おすすめ図書]